One-Credit Modular Statistical Methods Courses: 1977-1984
Preface
Ken Tiahrt came to my (Dick Lund's) office one early Monday morning sometime
in winter of 1977. He had spent the prior couple of days in Helena talking to persons
in the State government. Some there wanted to expand their knowledge about statistical
procedures. He told me "Dick, we need to develop some short courses for use off campus.
People in Helena want that and it could be an important outreach for our Statistical
Laboratory!"
Our conversation moved forward into the needs we saw at home in MSU, and the
possibility of separating our 300-level Statistical Methods courses into a collection
of short courses. I gave him an outline consisting of two‑credit short courses. But
no, he wanted smaller ones that perhaps could be delivered off‑campus in a two‑day
weekend, or on campus in little more than two‑weeks. We believed the MSU registrar
would never accept such, nor would it fit his computer‑driven publication of class
schedule listings. We might need a "Tiahrt work‑around" to create short courses. Ken
was now our Head of the Department of Mathematics!
But, surprisingly for us, Stuart E. Knapp, MSU Vice President for Academic
Affairs, liked trying our new idea! And, the result is the sequence of one‑credit
courses I taught that first summer in 1977. These are the courses listed in MSU Catalog 1978‑80. The one‑credit modules continued to be offered up to 1984. Then they were changed
into the set of two‑credit courses listed in MSU Catalog 1984‑86. Finally our Statistical
Methods reverted to term‑long courses, but at a 400 level, when MSU changed to a semester
system in 1991.
The following description is based on a report I wrote in winter 1981 for new
instructors, others on campus, or in particular, anyone Tiahrt wanted to send it to.
The report was an update of my invited presentation at the August, 1978, meeting of
the American Statistical Association, that being after having taught the sequence
two summers and one regular class year.
Return to Detailed Chronicle of the Statistics Program: 1977-1990
Modularized Instruction of Statistical Methods
by Richard E. Lund, Associate Professor of Statistics, Montana State University
For many years the Department of Mathematics at Montana State University offered a 300‑level sequence of three statistical methods courses for four credits each quarter. Enrollment consisted of one‑third undergraduate and two‑thirds graduate students. Programs served included agricultural, biological, social and physical sciences as well as statistics majors. Calculus was not a prerequisite.
The goals of the course sequence were (1) to introduce students to the statistical methods they were likely to encounter in their field of study and (2) to give them a sufficient understanding of the reasoning underlying the methods to use them wisely. Students were expected to acquire an ability to understand and evaluate statistical procedures used in the scientific literature in their field and to be able to communicate with a statistical consultant. The course was a prerequisite for thesis research data analysis for many students.
First quarter material focused on terminology, random sampling, elementary statistical inference but continued through attribute data analysis and linear regression. The second quarter continued through multiple regression and analysis of variance while the third quarter treated non‑parametric methods. Laboratory problems provided practice in applying the methods to realistic data and involved some computer data analysis.
The main deficiency of the course resulted from the diverse interests of the students. They preferred treatment of only the specific methods currently popular in their field. Students and advisors complained about both the excess in topics covered in some areas as well as the omissions and lack of depth in others. Students often failed to take the final quarter on non‑parametrics but requested some exposure to the topic.
Our solution to the problem was to develop fifteen self‑contained one credit mini‑courses or modules each entailing two and one‑half weeks classwork. The students and advisors could then select the specific topics appropriate for their field of study. The purpose of this paper is to describe and evaluate modularized instruction of statistical methods. The modular format was implemented summer quarter, 1977. The evaluation herein is based on two and one‑half years of operational experience.
Instruction Format
Each module is allocated ten classroom hours spread over two and one‑half weeks during non‑summer quarters. Eight modules are offered per quarter by means of a four module sequence in the morning and another in the afternoon. Table 1 specifies the topics currently taught. Table 2 shows the schedule for 1978‑79. Starting dates are selected to prevent university holidays from infringing on continuity for the ten classroom hours.

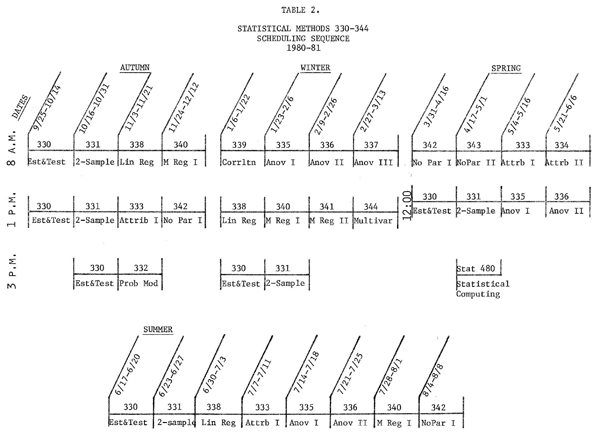
Seven of the ten assigned classroom hours are utilized for formal lecture. One or two hours are used in recitation, review and problem work. The remainder is associated with a final exam which is usually given in the evening in order to allow two hours work time.
Three laboratory homework problems are assigned in each module. A problem typically requires 4 to 6 hours student effort. It often consists of data representative of ongoing campus research. Each student is provided a unique computer-generated data set to illustrate the variability among observed data in real research. The resulting empirical measures of uncertainty (such as the class's type I and II error rates) are presented and discussed.
An interactive computer package is used for data analysis. Each lab problem requires some computer analysis and some hand calculator work. The students may be required to verify the computer analysis on simple data set examples, but generally they concentrate on organizing and directing the computer effort and interpreting output.
Lab problems are distributed prior to lecturing upon the material to which they pertain. About one week is allowed for completion and students are expected to work on their labs in conjunction with lecture and textbook study. An instructor-completed lab‑key based on one randomly-drawn data set is posted one day prior to lab due date. Students are expected to verify their own effort by comparison to the key. The proximity of the final exam usually requires immediate feedback on the students' data analysis effort. The students become familiar with the nature of statistical variability because the key will never agree exactly with their lab results. The instructor mainly checks the submitted lab reports for completeness and gross errors.
Example of Lab Problem for Modular Course 333

 (pdf version of the lab problem)
(pdf version of the lab problem)
An open‑book open‑notes final exam is used which emphasizes basic understanding and ability to apply the statistical methods treated in the module. Problems are often similar to those used in lab work. Calculation effort is minimized either by providing summarizing statistics or extremely simple data sets.
The currently assigned text, Snedecor and Cochran (1967), offers some material on nearly all fifteen modules. Dixon and Massey (1969) is used for supplementary reading in the introductory sections and offers several valuable tables not provided in the assigned text. Mosteller and Rourke (1973) is used for additional reading on non‑parametrics and Fleiss (1973) is used for attribute data analysis. Students are expected to purchase Snedecor and Cochran. A few copies of the others are made available for sale and copies are placed in library reserve.
The depth reached in the final module for most topics exceeds the textbooks noted above. Handouts on formulas, key ideas and examples are generally used for these modules rather than reading assignments in more advanced texts. Students have experienced difficulty in moving from one text to another and in understanding short segments of a text without background reading in the same text. The multivariate statistics module is handled entirely by lecture and handouts.
Course Administration
As noted earlier, these courses were set up to serve beginning graduate students and advanced undergraduates in all science disciplines of the university. The new format itself was initiated in response to needs expressed at a meeting of representatives from several campus disciplines. Consequently, information flow during the transition phase was a major problem which has not yet been solved completely. Students often are unable to decide which topics are best for their subject area. Considerable advising is required on the part of the statistical methods instructor.
The computer-based university registration procedure is not sufficiently flexible to handle the modular format. A special registration utilizing the university drop‑add procedure is used to "add" the modules desired. The mere processing of up to eight one‑credit courses per quarter increases the likelihood of errors.
A departmental policy was developed to accept new registrations in a module through the second day of class and allow drops through the seventh. Our add and drop restrictions differ greatly from normal university policy on drop‑adds.
Student Opinions
Student opinions were collected by questionnaires at mid‑year during the first and third years. The results below relating to proportions of students having certain opinions are based on the 35 students out of 41 enrolled in one or more modules at the time of collection.
Students liked the close proximity of the exam to classroom treatment of a topic and the limitations on the breadth of material on any one exam. But the rapidity of the lecture‑practice‑exam cycle also was a disadvantage for learning topics needing an "incubation" period. This problem has appeared most strongly in the introductory module which concerns random sampling, its implications, and one‑sample inference. Students were also just becoming acquainted with the modular format. Many students seem to require three-days attendance in a new course before they begin serious study. Consideration is now being given to expanding the length of the introductory module.
Nearly all students indicated a belief that they learned more under the new format than they would have expected to learn under the old format. A few indicated difficulties associated with the "choppiness" of the overall series and uneasiness caused by not proceeding sequentially through a single textbook. Transfer from one instructor to another for only 2 1/2 weeks was a noted problem for a few students.
About two‑thirds of the students were able to take some advantage of the flexibility for selecting modules they especially desired. The other one‑third were generally restricted by course scheduling problems.
Students' opinions were solicited concerning textbook usage. About one‑half the students reacted favorable to the multiple text concept, but the other one‑half was divided between being neutral and being dissatisfied. Many students thought they learned more by reading material from more than one author; however, others were apparently confused by the changes in symbols, formulas. tables and style of presentation.
Very favorable comments were received concerning the contribution of the lab problems. A few students wished the labs could be shortened and simplified, but an equal number thought they should be made even more detailed, realistic, and consequently time consuming. Many said they simply could not have learned the material, nor passed the exams, without the study‑aid‑homework aspect of the labs. Students especially wanted immediate feedback on their lab problems. This led to the policy of posting a lab‑key even before requiring the lab reports to be handed‑in. Students also wanted their lab reports evaluated in detail and weighted more heavily when determining the course grade. This suggestion appears to be impossible to fulfill in view of current instructor-time allocated to the course.
These opinions are of course quite subjective. The instructors certainly tried to overcome deficiencies in the new format and motivated students to circumvent obstacles. While the students overwhelmingly recommended continuance, they may also be reflecting only the positive thinking of the instructors.
Evaluations
My evaluation is that the new format enables students to learn more about the topics they select. Lab problems for the final module in most topics achieve considerably greater depth than previously attained. Exam performance is also significantly better.
Development and transition to the modular format of course required considerable instructor effort. But it now appears the modular format will require an additional one‑third in instructor time per credit when compared to the traditional format. The four‑fold change in registration and final grade assignment plus the associated additional student advising has surely doubled administrative effort. Four one‑credit modules utilize four two‑hour exams and twelve lab problems while the traditional four‑credit course used only two one‑hour exams, a two‑hour final and nine lab problems. The traditional format also provided the teaching efficiency associated with preparation of a single lecture for the two identical morning and afternoon course sections.
Our intention was to design nearly self‑contained modules with a minimum of prerequisites. Nevertheless. students who have omitted some of the more elementary modules have confronted handicaps. For example some students chose to enroll only in the introductory module and linear regression while their classmates enrolled in the comparison of two samples and the analysis of variance I modules which precede linear regression in the course sequence. The separation of total sum‑of‑squares into regression and residual sum‑of‑squares and development of the associated statistical tests were more easily grasped by the students who completed the analysis of variance I module. Tests for equality of slopes for two regressions were more quickly learned by students who took the two‑sample module. Students omitting modules in the sequences scheduled in Table 2 are now advised of a need for extra reading and study, and that selection flexibility requires compensatory student effort.
Comments received from faculty in other disciplines have been universally favorable. They especially like the possibility for a student to obtain additional coursework on a statistical method when the need becomes apparent in his/her thesis research. They have expressed difficulty in deciding which modules to advise their students to take.
Material Availability (in 1981)
A brief description of the interactive computer package used to analyze lab problems has been submitted for publication. Program source is written in a transportable Fortran and is available for purchase. A copy of the fifteen mini‑course outlines, hand‑outs and lab problems (about 110 pages) will be supplied on request to the author for $15. Completion of a proprietary agreement will be required before this material can be duplicated for classroom use.
References
Dixon, Wilfrid J. and Massey, Frank J. Jr. (19691, Introduction to Statistical Analysis, New York: McGraw‑Hill Book Company.
Fleiss, Joseph L. (1973), Statistical Methods for Rates and Proportions, New York: John Wiley & Sons.
Mosteller, Frederick and Rourke, Robert E. (1973), Sturdy Statistics, Reading, Massachusetts: Addison‑Wesley Publishing Company.
Snedecor, George W. and Cochran, William G. (1967), Statistical Methods, Ames, Iowa: Iowa State University Press.
Return to Detailed Chronicle of the Statistics Program: 1977-1990
Next: Era 8 (Stat Program)
Table of Contents
Last revised: 2020-07-14
